
But what about the third altitude of a right triangle? The other leg of the right triangle is the altitude of the equilateral triangle, so solve using the Pythagorean Theorem:Ī = 20.7846 y d s a=20.7846 yds a = 20.7846 y d sĪnytime you can construct an altitude that cuts your original triangle into two right triangles, Pythagoras will do the trick! Use the Pythagorean Theorem for finding all altitudes of all equilateral and isosceles triangles.įor right triangles, two of the altitudes of a right triangle are the legs themselves. You only need to know its altitude.Ĭonstructing an altitude from any base divides the equilateral triangle into two right triangles, each one of which has a hypotenuse equal to the original equilateral triangle's side, and a leg 1 2 \frac 2 1 that length. It will have three congruent altitudes, so no matter which direction you put that in a shipping box, it will fit. Imagine △ E Q U \triangle EQU △ EQ U with sides labeled 24 yards. What about an equilateral triangle, with three congruent sides and three congruent angles. Not every triangle is as fussy as a scalene, obtuse triangle. What about the other two altitudes? If you insisted on using side GU ( ∠ D \angle D ∠ D) for the altitude, you would need a box 9.37 cm tall, and if you rotated the triangle to use side DG ( ∠ U \angle U ∠ U), your altitude there is 7.56 cm tall. You would naturally pick the altitude or height that allowed you to ship your triangle in the smallest rectangular carton, so you could stack a lot on a shelf.Īltitude for side UD ( ∠ G \angle G ∠ G) is only 4.3 cm. Think of building and packing triangles again. Obtuse triangles - One interior angle is obtuse, or greater than 90°Įvery triangle has three altitudes. Oblique triangles break down into two types:Īcute triangles - All interior angles are acute, or each less than 90° Right - One right angle ( 90°) and two acute angles Anglesīy their interior angles, triangles have other classifications:

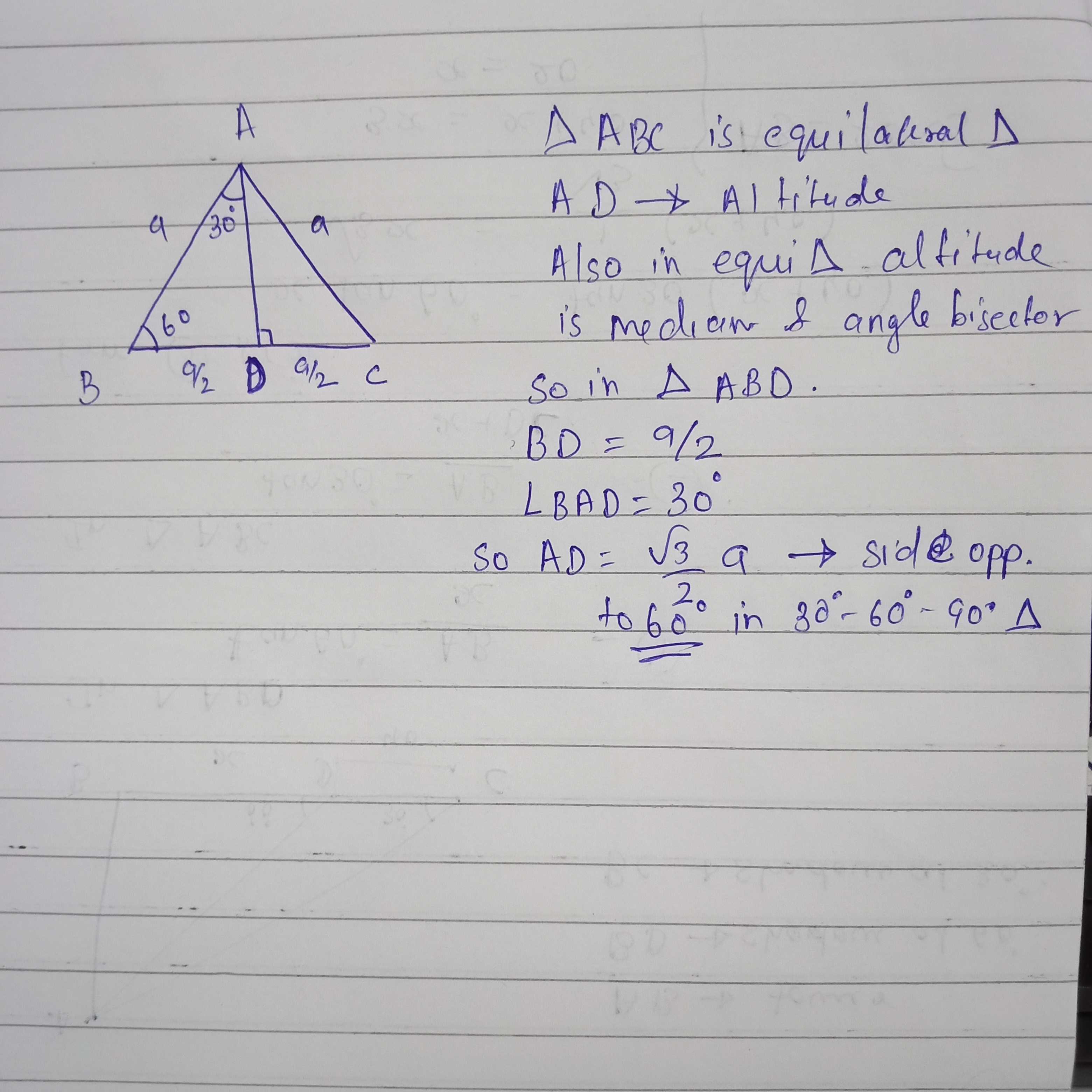
Most mathematicians agree that the classic equilateral triangle can also be considered an isosceles triangle, because an equilateral triangle has two congruent sides. Scalene - No two sides are congruent (equal in length) By their sides, you can break them down like this: Sides You can classify triangles either by their sides or their angles. A triangle gets its name from its three interior angles. To find the altitude, we first need to know what kind of triangle we are dealing with.
To get the altitude for ∠ D \angle D ∠ D, you must extend the side GU far past the triangle and construct the altitude far to the right of the triangle. To get that altitude, you need to project a line from side DG out very far past the left of the triangle itself. The altitude from ∠ G \angle G ∠ G drops down and is perpendicular to UD, but what about the altitude for ∠ U \angle U ∠ U? Label the sides too side GU=17 cm, UD=37 cm, and DG=21 cm.įor △ G U D \triangle GUD △ G U D, no two sides are equal and one angle is greater than 90°, so you know you have a scalene, obtuse (oblique) triangle. We can construct three different altitudes, one from each vertex.ĭraw a scalene △GUD with ∠G=154°, ∠U=14.8°, and ∠D=11.8°. Here is scalene △ G U D \triangle GUD △ G U D. The height or altitude of a triangle depends on which base you use for a measurement.

How big a rectangular box would you need? Your triangle has length, but what is its height? Imagine you ran a business making and sending out triangles, and each had to be put in a rectangular cardboard shipping carton. An altitude is a line drawn from a triangle's vertex down to the opposite base, so that the constructed line is perpendicular to the base.


 0 kommentar(er)
0 kommentar(er)
